Algebra
Calculus
Trigonometry
Matrix
Question
Solve the equation
x1=2−32,x2=2+32
Alternative Form
x1≈0.740079,x2≈3.259921
Evaluate
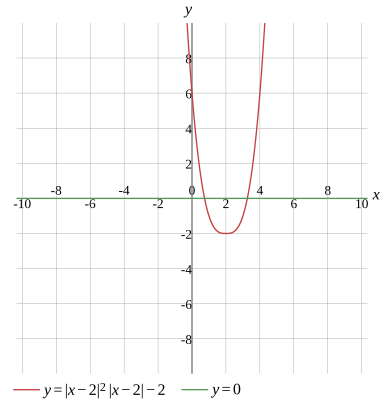
∣x−2∣2∣x−2∣−2=0
Evaluate the power
(x−2)2∣x−2∣−2=0
Separate the equation into 2 possible cases
(x−2)2(x−2)−2=0,x−2≥0(x−2)2(−(x−2))−2=0,x−2<0
Solve the equation
More Steps
Evaluate
(x−2)2(x−2)−2=0
Calculate
More Steps
Evaluate
(x−2)2(x−2)−2
Expand the expression
x3−6x2+12x−8−2
Subtract the numbers
x3−6x2+12x−10
x3−6x2+12x−10=0
Calculate
x=2+32
x=2+32,x−2≥0(x−2)2(−(x−2))−2=0,x−2<0
Solve the inequality
More Steps
Evaluate
x−2≥0
Move the constant to the right side
x≥0+2
Removing 0 doesn't change the value,so remove it from the expression
x≥2
x=2+32,x≥2(x−2)2(−(x−2))−2=0,x−2<0
Solve the equation
More Steps
Evaluate
(x−2)2(−(x−2))−2=0
Calculate
(x−2)2(−x+2)−2=0
Calculate
More Steps
Evaluate
(x−2)2(−x+2)−2
Expand the expression
−x3+6x2−12x+8−2
Subtract the numbers
−x3+6x2−12x+6
−x3+6x2−12x+6=0
Calculate
x=2−32
x=2+32,x≥2x=2−32,x−2<0
Solve the inequality
More Steps
Evaluate
x−2<0
Move the constant to the right side
x<0+2
Removing 0 doesn't change the value,so remove it from the expression
x<2
x=2+32,x≥2x=2−32,x<2
Find the intersection
x=2+32x=2−32,x<2
Find the intersection
x=2+32x=2−32
Solution
x1=2−32,x2=2+32
Alternative Form
x1≈0.740079,x2≈3.259921
Show Solution
Graph
