Algebra
Calculus
Trigonometry
Matrix
Question
Solve the equation
x=20187
Alternative Form
x=9,35
Evaluate
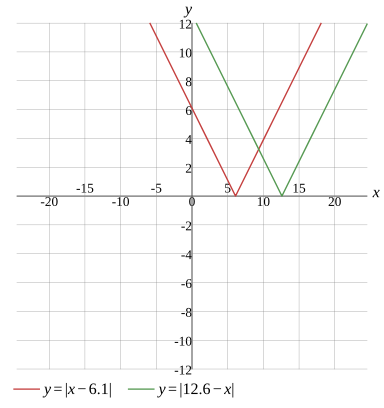
∣x−6,1∣=∣12,6−x∣
Simplify
x−1061=∣12,6−x∣
Simplify
x−1061=563−x
Evaluate
x−1061=563−xx−1061=−(563−x)
Calculate
More Steps
Evaluate
x−1061=563−x
Multiply both sides of the equation by LCM
(x−1061)×10=(563−x)×10
Calculate
More Steps
Evaluate
(x−1061)×10
Apply the distributive property
x×10−1061×10
Use the commutative property to reorder the terms
10x−1061×10
Multiply the numbers
10x−61
10x−61=(563−x)×10
Calculate
More Steps
Evaluate
(563−x)×10
Apply the distributive property
563×10−x×10
Multiply the numbers
126−x×10
Use the commutative property to reorder the terms
126−10x
10x−61=126−10x
Move the expression to the left side
10x−61+10x=126
Move the expression to the right side
10x+10x=126+61
Add and subtract
More Steps
Evaluate
10x+10x
Collect like terms by calculating the sum or difference of their coefficients
(10+10)x
Add the numbers
20x
20x=126+61
Add and subtract
20x=187
Divide both sides
2020x=20187
Divide the numbers
x=20187
x=20187x−1061=−(563−x)
Calculate
More Steps
Evaluate
x−1061=−(563−x)
Calculate
x−1061=−563+x
Cancel equal terms on both sides of the expression
−1061=−563
Calculate
−6,1=−563
Calculate
−6,1=−12,6
The statement is false for any value of x
x∈∅
x=20187x∈∅
Solution
x=20187
Alternative Form
x=9,35
Show Solution
Graph
