Solve (x^2)^2=(1-x)^2 - ScanMath

HOME CALCULATOR

DOWNLOAD FOR FREE

Algebra
Calculus
Trigonometry
Matrix

Type your math problem... (x^2)^2=(1-x)^2

Question

Solve the equation

x_{1} = - \frac{1 + 5}{2}, x_{2} = \frac{- 1 + 5}{2}

Alternative Form

x_{1} \approx - 1.618034, x_{2} \approx 0.618034

Evaluate

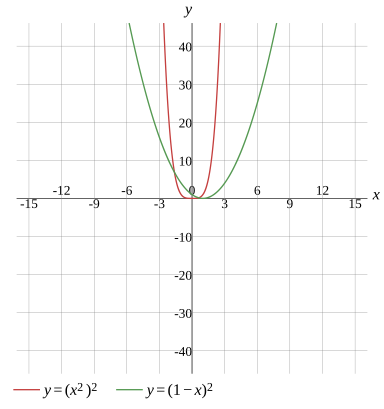
(x^{2})^{2} = (1 - x)^{2}

Simplify

More Steps

x^{4} = (1 - x)^{2}

Raise both sides of the equation to the reciprocal of the exponent

(x^{4})^{\frac{1}{2}} = ((1 - x)^{2})^{\frac{1}{2}}

Evaluate the power

x^{2} = ∣ 1 - x ∣

Swap the sides

∣ 1 - x ∣ = x^{2}

Rewrite the expression

∣ 1 - x ∣ - x^{2} = 0

Separate the equation into 2 possible cases

1 - x - x^{2} = 0, 1 - x \geq 0 - (1 - x) - x^{2} = 0, 1 - x < 0

Solve the equation

More Steps

x = \frac{- 1 + 5}{2} x = - \frac{1 + 5}{2}, 1 - x \geq 0 - (1 - x) - x^{2} = 0, 1 - x < 0

Solve the inequality

More Steps

x = \frac{- 1 + 5}{2} x = - \frac{1 + 5}{2}, x \leq 1 - (1 - x) - x^{2} = 0, 1 - x < 0

Solve the equation

More Steps

x = \frac{- 1 + 5}{2} x = - \frac{1 + 5}{2}, x \leq 1 x \in / R, 1 - x < 0

Solve the inequality

More Steps

x = \frac{- 1 + 5}{2} x = - \frac{1 + 5}{2}, x \leq 1 x \in / R, x > 1

Find the intersection

x = \frac{- 1 + 5}{2} x = - \frac{1 + 5}{2} x \in / R, x > 1

Find the intersection

x = \frac{- 1 + 5}{2} x = - \frac{1 + 5}{2} x \in / R

Find the union

x = \frac{- 1 + 5}{2} x = - \frac{1 + 5}{2}

Solution

x_{1} = - \frac{1 + 5}{2}, x_{2} = \frac{- 1 + 5}{2}

Alternative Form

x_{1} \approx - 1.618034, x_{2} \approx 0.618034

Show Solution

Graph

Graph