Solve 3(x^2)=2(2-x)

Question

Solve the quadratic equation

Solve using the quadratic formula

Solve by completing the square

Solve using the PQ formula

x_{1} = - \frac{1 + 13}{3}, x_{2} = \frac{- 1 + 13}{3}

Alternative Form

x_{1} \approx - 1.535184, x_{2} \approx 0.868517

Evaluate

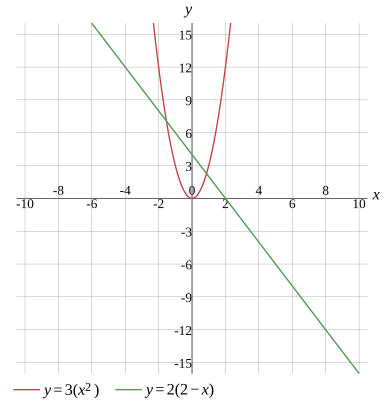
3 x^{2} = 2 (2 - x)

Expand the expression

More Steps

3 x^{2} = 4 - 2 x

Move the expression to the left side

3 x^{2} - 4 + 2 x = 0

Rewrite in standard form

3 x^{2} + 2 x - 4 = 0

Substitute a= 3,b= 2 and c= - 4 into the quadratic formula x = \frac{- b \pm b ^{2} - 4 a c}{2 a}

x = \frac{- 2 \pm 2 ^{2} - 4 \times 3 ( - 4 )}{2 \times 3}

Simplify the expression

x = \frac{- 2 \pm 2 ^{2} - 4 \times 3 ( - 4 )}{6}

Simplify the expression

More Steps

x = \frac{- 2 \pm 52}{6}

Simplify the radical expression

More Steps

x = \frac{- 2 \pm 2 13}{6}

Separate the equation into 2 possible cases

x = \frac{- 2 + 2 13}{6} x = \frac{- 2 - 2 13}{6}

Simplify the expression

More Steps

x = \frac{- 1 + 13}{3} x = \frac{- 2 - 2 13}{6}

Simplify the expression

More Steps

x = \frac{- 1 + 13}{3} x = - \frac{1 + 13}{3}

Solution

x_{1} = - \frac{1 + 13}{3}, x_{2} = \frac{- 1 + 13}{3}

Alternative Form

x_{1} \approx - 1.535184, x_{2} \approx 0.868517

Show Solution