Solve 5 - |1/2x - 4| = 2 1/2

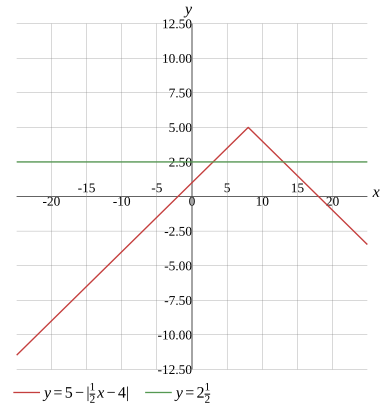
Question

Solve the equation

x_{1} = 3, x_{2} = 13

Evaluate

5 - \frac{1}{2} x - 4 = 2 \frac{1}{2}

Covert the mixed number to an improper fraction

More Steps

5 - \frac{1}{2} x - 4 = \frac{5}{2}

Move the constant to the right-hand side and change its sign

- \frac{1}{2} x - 4 = \frac{5}{2} - 5

Subtract the numbers

More Steps

- \frac{1}{2} x - 4 = - \frac{5}{2}

Calculate

\frac{1}{2} x - 4 = \frac{5}{2}

Separate the equation into 2 possible cases

\frac{1}{2} x - 4 = \frac{5}{2} \frac{1}{2} x - 4 = - \frac{5}{2}

Solve the equation for x

More Steps

x = 13 \frac{1}{2} x - 4 = - \frac{5}{2}

Solve the equation for x

More Steps

x = 13 x = 3

Solution

x_{1} = 3, x_{2} = 13

Show Solution