Algebra
Calculus
Trigonometry
Matrix
Question
Function
Evaluate the derivative
Find the domain
Find the c-intercept/zero
Load more
f′(c)=9c2−36c+27
Evaluate
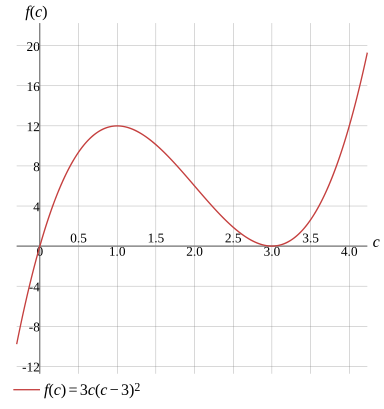
f(c)=3c(c−3)2
Take the derivative of both sides
f′(c)=dcd(3c(c−3)2)
Use differentiation rule dxd(f(x)×g(x))=dxd(f(x))×g(x)+f(x)×dxd(g(x))
f′(c)=dcd(3c)×(c−3)2+3c×dcd((c−3)2)
Calculate
More Steps
Calculate
dcd(3c)
Use differentiation rule dxd(cf(x))=c×dxd(f(x))
3×dcd(c)
Use dxdxn=nxn−1 to find derivative
3×1
Any expression multiplied by 1 remains the same
3
f′(c)=3(c−3)2+3c×dcd((c−3)2)
Calculate
More Steps
Calculate
dcd((c−3)2)
Use the chain rule dxd(f(g))=dgd(f(g))×dxd(g) where the g=c−3, to find the derivative
dgd(g2)×dcd(c−3)
Use dxdxn=nxn−1 to find derivative
2g×dcd(c−3)
Calculate
2g×1
Substitute back
2(c−3)×1
Rewrite the expression
2(c−3)
Apply the distributive property
2c−2×3
Multiply the numbers
2c−6
f′(c)=3(c−3)2+3c(2c−6)
Calculate
f′(c)=3(c−3)2+6c2−18c
Simplify
More Steps
Evaluate
3(c−3)2
Simplify
3(c2−6c+9)
Apply the distributive property
3c2+3(−6c)+3×9
Multiply the terms
3c2−18c+3×9
Multiply the terms
3c2−18c+27
f′(c)=3c2−18c+27+6c2−18c
Solution
More Steps
Evaluate
3c2−18c+27+6c2−18c
Add the terms
9c2−18c+27−18c
Subtract the terms
9c2−36c+27
f′(c)=9c2−36c+27
Show Solution
Graph
