Question
Function
Evaluate the derivative
Find the domain
Find the θ-intercept/zero
Load more

r′=4sin(θ)
Evaluate
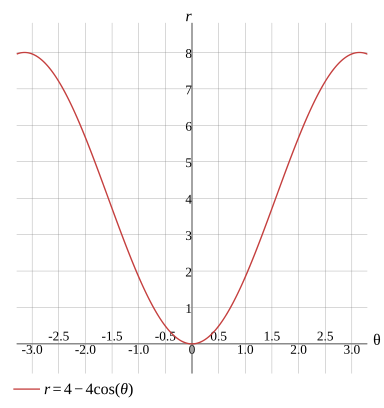
r=4−4cos(θ)
Take the derivative of both sides
r′=dθd(4−4cos(θ))
Use differentiation rule dxd(f(x)±g(x))=dxd(f(x))±dxd(g(x))
r′=dθd(4)−dθd(4cos(θ))
Use dxd(c)=0 to find derivative
r′=0−dθd(4cos(θ))
Calculate
More Steps


Calculate
dθd(4cos(θ))
Simplify
4×dθd(cos(θ))
Use dxd(cosx)=−sinx to find derivative
4(−sin(θ))
Calculate
−4sin(θ)
r′=0−(−4sin(θ))
Solution
r′=4sin(θ)
Show Solution

Rewrite the equation
16y2=x4+y4+8x3+8xy2+2x2y2
Evaluate
r=4−4cos(θ)
Multiply both sides
r2=4r−4cos(θ)×r
Rewrite the expression
4cos(θ)×r+r2−4r=0
Use substitution
More Steps


Evaluate
4cos(θ)×r+r2−4r
Use substitution
More Steps


Evaluate
4cos(θ)×r
Use the commutative property to reorder the terms
rcos(θ)×4
To covert the equation to rectangular coordinates using conversion formulas,substitute rcosθ for x
x×4
Multiply the terms
4x
4x+r2−4r
To covert the equation to rectangular coordinates using conversion formulas,substitute x2+y2 for r2
4x+x2+y2−4r
4x+x2+y2−4r=0
Simplify the expression
−4r=−4x−x2−y2
Square both sides of the equation
(−4r)2=(−4x−x2−y2)2
Evaluate
16r2=(−4x−x2−y2)2
To covert the equation to rectangular coordinates using conversion formulas,substitute x2+y2 for r2
16(x2+y2)=(−4x−x2−y2)2
Evaluate the power
16(x2+y2)=(4x+x2+y2)2
Calculate
16x2+16y2=16x2+x4+y4+8x3+8xy2+2x2y2
Move the expression to the left side
16x2+16y2−16x2=x4+y4+8x3+8xy2+2x2y2
Calculate
0+16y2=x4+y4+8x3+8xy2+2x2y2
Solution
16y2=x4+y4+8x3+8xy2+2x2y2
Show Solution

Graph