Question
Function
Find the inverse
Evaluate the derivative
Find the domain
Load more

f−1(θ)=2arccos(21θ)
Evaluate
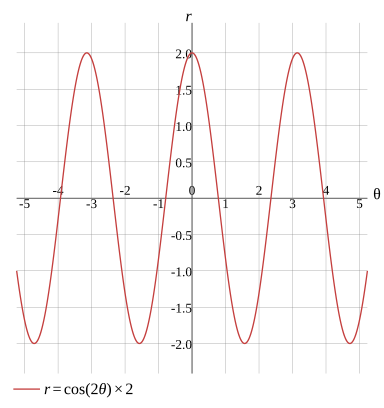
r=cos(2θ)×2
Simplify
r=2cos(2θ)
Interchange θ and y
θ=2cos(2y)
Calculate
More Steps


Evaluate
θ=2cos(2y)
Swap the sides of the equation
2cos(2y)=θ
Multiply both sides of the equation by 21
2cos(2y)×21=θ×21
Calculate
cos(2y)=θ×21
Calculate
cos(2y)=21θ
Use the inverse trigonometric function
2y=arccos(21θ)
Divide both sides
22y=2arccos(21θ)
Divide the numbers
y=2arccos(21θ)
y=2arccos(21θ)
Solution
f−1(θ)=2arccos(21θ)
Show Solution

Solve the equation
Solve for θ
Solve for r
θ=2arccos(21r)
Evaluate
r=cos(2θ)×2
Simplify
r=2cos(2θ)
Swap the sides of the equation
2cos(2θ)=r
Multiply both sides of the equation by 21
2cos(2θ)×21=r×21
Calculate
cos(2θ)=r×21
Calculate
cos(2θ)=21r
Use the inverse trigonometric function
2θ=arccos(21r)
Divide both sides
22θ=2arccos(21r)
Solution
θ=2arccos(21r)
Show Solution

Rewrite the equation
x6+3x4y2+3x2y4+y6=4x4−8x2y2+4y4
Evaluate
r=cos(2θ)×2
Simplify
r=2cos(2θ)
Simplify the expression
r=2cos2(θ)−2sin2(θ)
Multiply both sides
r3=2(rcos(θ))2−2(rsin(θ))2
Rewrite the expression
−2(rcos(θ))2+2(rsin(θ))2+r3=0
Use substitution
More Steps


Evaluate
−2(rcos(θ))2+2(rsin(θ))2+r3
To covert the equation to rectangular coordinates using conversion formulas,substitute rcosθ for x
−2x2+2(rsin(θ))2+r3
To covert the equation to rectangular coordinates using conversion formulas,substitute rsinθ for y
−2x2+2y2+r3
−2x2+2y2+r3=0
Simplify the expression
r3=2x2−2y2
Evaluate
r2×r=2x2−2y2
Evaluate
(x2+y2)r=2x2−2y2
Square both sides of the equation
((x2+y2)r)2=(2x2−2y2)2
Evaluate
(x2+y2)2r2=(2x2−2y2)2
To covert the equation to rectangular coordinates using conversion formulas,substitute x2+y2 for r2
(x2+y2)2(x2+y2)=(2x2−2y2)2
Use substitution
(x2+y2)3=(2x2−2y2)2
Solution
x6+3x4y2+3x2y4+y6=4x4−8x2y2+4y4
Show Solution

Graph