Algebra
Calculus
Trigonometry
Matrix
Question
Solve the equation
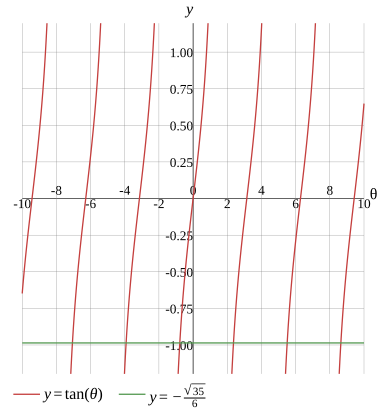
θ=arctan(−635)+kπ,k∈Z
Alternative Form
θ≈−44.596495∘+180∘k,k∈Z
Alternative Form
θ≈−0.778356+kπ,k∈Z
Evaluate
tan(θ)=−635
Find the domain
tan(θ)=−635,θ=2π+kπ,k∈Z
Use the inverse trigonometric function
θ=arctan(−635)
Add the period of kπ,k∈Z to find all solutions
θ=arctan(−635)+kπ,k∈Z
Check if the solution is in the defined range
θ=arctan(−635)+kπ,k∈Z,θ=2π+kπ,k∈Z
Solution
θ=arctan(−635)+kπ,k∈Z
Alternative Form
θ≈−44.596495∘+180∘k,k∈Z
Alternative Form
θ≈−0.778356+kπ,k∈Z
Show Solution
Rewrite the equation
6y=−35×x
Evaluate
tan(θ)=−635
Multiply both sides of the equation by LCD
tan(θ)×6=−635×6
Simplify the equation
6tan(θ)=−635×6
Simplify the equation
6tan(θ)=−35
Rewrite the expression
0=−6tan(θ)−35
Use substitution
0=−x6y−35
Multiply both sides of the equation by LCD
0×x=(−x6y−35)x
Any expression multiplied by 0 equals 0
0=(−x6y−35)x
Simplify the equation
More Steps
Evaluate
(−x6y−35)x
Apply the distributive property
−x6y×x−35×x
Simplify
−6y−35×x
0=−6y−35×x
Move the expression to the left side
0−(−6y)=−35×x
Solution
6y=−35×x
Show Solution
Graph
