Solve x^2+y-3\sqrt x^22=1

Question

Function

Find the x -intercept/zero

Find the y-intercept

x_{1} = 3 - 10, x_{2} = 3 + 10

Evaluate

x^{2} + y - 3 (x)^{2} \times 2 = 1

To find the x -intercept,set y =0

x^{2} + 0 - 3 (x)^{2} \times 2 = 1

Simplify

More Steps

Evaluate

x^{2} + 0 - 3 (x)^{2} \times 2

Multiply

More Steps

Multiply the terms

- 3 (x)^{2} \times 2

Multiply the terms

- 6 (x)^{2}

Multiply the terms

- 6 x

x^{2} + 0 - 6 x

Removing 0 doesn't change the value,so remove it from the expression

x^{2} - 6 x

x^{2} - 6 x = 1

Move the expression to the left side

x^{2} - 6 x - 1 = 0

Substitute a= 1,b= - 6 and c= - 1 into the quadratic formula x = \frac{- b \pm b ^{2} - 4 a c}{2 a}

x = \frac{6 \pm ( - 6 ) ^{2} - 4 ( - 1 )}{2}

Simplify the expression

More Steps

Evaluate

(- 6)^{2} - 4 (- 1)

Simplify

(- 6)^{2} - (- 4)

Rewrite the expression

6^{2} - (- 4)

If a negative sign or a subtraction symbol appears outside parentheses, remove the parentheses and change the sign of every term within the parentheses

6^{2} + 4

Evaluate the power

36 + 4

Add the numbers

40

x = \frac{6 \pm 40}{2}

Simplify the radical expression

More Steps

Evaluate

40

Write the expression as a product where the root of one of the factors can be evaluated

4 \times 10

Write the number in exponential form with the base of 2

2^{2} \times 10

The root of a product is equal to the product of the roots of each factor

2^{2} \times 10

Reduce the index of the radical and exponent with 2

210

x = \frac{6 \pm 2 10}{2}

Separate the equation into 2 possible cases

x = \frac{6 + 2 10}{2} x = \frac{6 - 2 10}{2}

Simplify the expression

More Steps

Evaluate

x = \frac{6 + 2 10}{2}

Divide the terms

More Steps

Evaluate

\frac{6 + 2 10}{2}

Rewrite the expression

\frac{2 ( 3 + 10 )}{2}

Reduce the fraction

3 + 10

x = 3 + 10

x = 3 + 10 x = \frac{6 - 2 10}{2}

Simplify the expression

More Steps

Evaluate

x = \frac{6 - 2 10}{2}

Divide the terms

More Steps

Evaluate

\frac{6 - 2 10}{2}

Rewrite the expression

\frac{2 ( 3 - 10 )}{2}

Reduce the fraction

3 - 10

x = 3 - 10

x = 3 + 10 x = 3 - 10

Solution

x_{1} = 3 - 10, x_{2} = 3 + 10

Show Solution

Solve the equation

Solve for x

Solve for y

x = 3 + 10 - y x = 3 - 10 - y

Evaluate

x^{2} + y - 3 (x)^{2} \times 2 = 1

Multiply

More Steps

Evaluate

- 3 (x)^{2} \times 2

Multiply the terms

- 6 (x)^{2}

Multiply the terms

- 6 x

x^{2} + y - 6 x = 1

Move the expression to the left side

x^{2} + y - 6 x - 1 = 0

Simplify

x^{2} + y - 1 - 6 x = 0

Rewrite in standard form

x^{2} - 6 x + y - 1 = 0

Substitute a= 1,b= - 6 and c= y - 1 into the quadratic formula x = \frac{- b \pm b ^{2} - 4 a c}{2 a}

x = \frac{6 \pm ( - 6 ) ^{2} - 4 ( y - 1 )}{2}

Simplify the expression

More Steps

Evaluate

(- 6)^{2} - 4 (y - 1)

Apply the distributive property

(- 6)^{2} - (4 y - 4)

Rewrite the expression

6^{2} - (4 y - 4)

If a negative sign or a subtraction symbol appears outside parentheses, remove the parentheses and change the sign of every term within the parentheses

6^{2} - 4 y + 4

Evaluate the power

36 - 4 y + 4

Add the numbers

40 - 4 y

x = \frac{6 \pm 40 - 4 y}{2}

Simplify the radical expression

More Steps

Evaluate

40 - 4 y

Factor the expression

4 (10 - y)

The root of a product is equal to the product of the roots of each factor

4 \times 10 - y

Evaluate the root

More Steps

Evaluate

4

Write the number in exponential form with the base of 2

2^{2}

Reduce the index of the radical and exponent with 2

2

2 10 - y

x = \frac{6 \pm 2 10 - y}{2}

Separate the equation into 2 possible cases

x = \frac{6 + 2 10 - y}{2} x = \frac{6 - 2 10 - y}{2}

Simplify the expression

More Steps

Evaluate

x = \frac{6 + 2 10 - y}{2}

Divide the terms

More Steps

Evaluate

\frac{6 + 2 10 - y}{2}

Rewrite the expression

\frac{2 ( 3 + 10 - y )}{2}

Reduce the fraction

3 + 10 - y

x = 3 + 10 - y

x = 3 + 10 - y x = \frac{6 - 2 10 - y}{2}

Solution

More Steps

Evaluate

x = \frac{6 - 2 10 - y}{2}

Divide the terms

More Steps

Evaluate

\frac{6 - 2 10 - y}{2}

Rewrite the expression

\frac{2 ( 3 - 10 - y )}{2}

Reduce the fraction

3 - 10 - y

x = 3 - 10 - y

x = 3 + 10 - y x = 3 - 10 - y

Show Solution

Testing for symmetry

Testing for symmetry about the origin

Testing for symmetry about the x-axis

Testing for symmetry about the y-axis

Not symmetry with respect to the origin

Evaluate

x^{2} + y - 3 x^{2} 2 = 1

Simplify the expression

x^{2} + y - 6 x = 1

To test if the graph of x^{2} + y - 6 x = 1 is symmetry with respect to the origin,substitute -x for x and -y for y

(- x)^{2} - y - 6 (- x) = 1

Evaluate

More Steps

Evaluate

(- x)^{2} - y - 6 (- x)

Multiply the numbers

(- x)^{2} - y + 6 x

Rewrite the expression

x^{2} - y + 6 x

x^{2} - y + 6 x = 1

Solution

Not symmetry with respect to the origin

Show Solution

Identify the conic

Find the standard equation of the parabola

Find the vertex of the parabola

Find the focus of the parabola

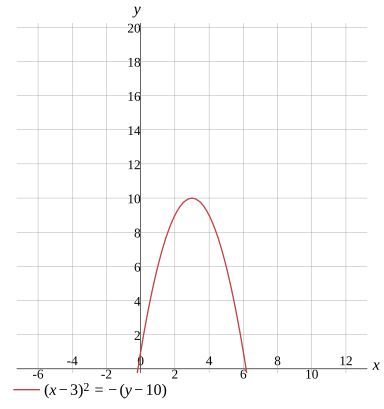
(x - 3)^{2} = - (y - 10)

Evaluate

x^{2} + y - 3 (x)^{2} \times 2 = 1

Calculate

x^{2} + y - 6 x = 1

Move the expression to the right-hand side and change its sign

x^{2} - 6 x = 1 - y

Use the commutative property to reorder the terms

x^{2} - 6 x = - y + 1

To complete the square, the same value needs to be added to both sides

x^{2} - 6 x + 9 = - y + 1 + 9

Use a^{2} - 2 ab + b^{2} = (a - b)^{2} to factor the expression

(x - 3)^{2} = - y + 1 + 9

Add the numbers

(x - 3)^{2} = - y + 10

Solution

(x - 3)^{2} = - (y - 10)

Show Solution

Rewrite the equation

r = \frac{- sin ( θ ) + 6 cos ( θ ) + 1 + 39 cos ^{2} ( θ ) - 6 sin ( 2 θ )}{2 cos ^{2} ( θ )} r = \frac{- sin ( θ ) + 6 cos ( θ ) - 1 + 39 cos ^{2} ( θ ) - 6 sin ( 2 θ )}{2 cos ^{2} ( θ )}

Evaluate

x^{2} + y - 3 (x)^{2} \times 2 = 1

Evaluate

More Steps

Evaluate

x^{2} + y - 3 (x)^{2} \times 2

Multiply

More Steps

Evaluate

- 3 (x)^{2} \times 2

Multiply the terms

- 6 (x)^{2}

Multiply the terms

- 6 x

x^{2} + y - 6 x

x^{2} + y - 6 x = 1

To convert the equation to polar coordinates,substitute x for r cos (θ) and y for r sin (θ)

(cos (θ) \times r)^{2} + sin (θ) \times r - 6 cos (θ) \times r = 1

Factor the expression

cos^{2} (θ) \times r^{2} + (sin (θ) - 6 cos (θ)) r = 1

Subtract the terms

cos^{2} (θ) \times r^{2} + (sin (θ) - 6 cos (θ)) r - 1 = 1 - 1

Evaluate

cos^{2} (θ) \times r^{2} + (sin (θ) - 6 cos (θ)) r - 1 = 0

Solve using the quadratic formula

r = \frac{- sin ( θ ) + 6 cos ( θ ) \pm ( sin ( θ ) - 6 cos ( θ ) ) ^{2} - 4 cos ^{2} ( θ ) ( - 1 )}{2 cos ^{2} ( θ )}

Simplify

r = \frac{- sin ( θ ) + 6 cos ( θ ) \pm 1 + 39 cos ^{2} ( θ ) - 6 sin ( 2 θ )}{2 cos ^{2} ( θ )}

Solution

r = \frac{- sin ( θ ) + 6 cos ( θ ) + 1 + 39 cos ^{2} ( θ ) - 6 sin ( 2 θ )}{2 cos ^{2} ( θ )} r = \frac{- sin ( θ ) + 6 cos ( θ ) - 1 + 39 cos ^{2} ( θ ) - 6 sin ( 2 θ )}{2 cos ^{2} ( θ )}

Show Solution

Find the first derivative

Find the derivative with respect to x

Find the derivative with respect to y

\frac{d y}{d x} = - 2 x + 6

Calculate

x^{2} + y - 3 x^{2} 2 = 1

Simplify the expression

x^{2} + y - 6 x = 1

Take the derivative of both sides

\frac{d}{d x} (x^{2} + y - 6 x) = \frac{d}{d x} (1)

Calculate the derivative

More Steps

Evaluate

\frac{d}{d x} (x^{2} + y - 6 x)

Use differentiation rules

\frac{d}{d x} (x^{2}) + \frac{d}{d x} (y) + \frac{d}{d x} (- 6 x)

Use \frac{d}{d x} x^{n} = n x^{n - 1} to find derivative

2 x + \frac{d}{d x} (y) + \frac{d}{d x} (- 6 x)

Evaluate the derivative

More Steps

Evaluate

\frac{d}{d x} (y)

Use differentiation rules

\frac{d}{d y} (y) \times \frac{d y}{d x}

Use \frac{d}{d x} x^{n} = n x^{n - 1} to find derivative

\frac{d y}{d x}

2 x + \frac{d y}{d x} + \frac{d}{d x} (- 6 x)

Evaluate the derivative

More Steps

Evaluate

\frac{d}{d x} (- 6 x)

Use differentiation rule \frac{d}{d x} (c f (x)) = c \times \frac{d}{d x} (f (x))

- 6 \times \frac{d}{d x} (x)

Use \frac{d}{d x} x^{n} = n x^{n - 1} to find derivative

- 6 \times 1

Any expression multiplied by 1 remains the same

- 6

2 x + \frac{d y}{d x} - 6

2 x + \frac{d y}{d x} - 6 = \frac{d}{d x} (1)

Calculate the derivative

2 x + \frac{d y}{d x} - 6 = 0

Move the expression to the right-hand side and change its sign

\frac{d y}{d x} = 0 - (2 x - 6)

Solution

More Steps

Evaluate

0 - (2 x - 6)

If a negative sign or a subtraction symbol appears outside parentheses, remove the parentheses and change the sign of every term within the parentheses

0 - 2 x + 6

Removing 0 doesn't change the value,so remove it from the expression

- 2 x + 6

\frac{d y}{d x} = - 2 x + 6

Show Solution

Find the second derivative

Find the second derivative with respect to x

Find the second derivative with respect to y

\frac{d ^{2} y}{d x ^{2}} = - 2

Calculate

x^{2} + y - 3 x^{2} 2 = 1

Simplify the expression

x^{2} + y - 6 x = 1

Take the derivative of both sides

\frac{d}{d x} (x^{2} + y - 6 x) = \frac{d}{d x} (1)

Calculate the derivative

More Steps

Evaluate

\frac{d}{d x} (x^{2} + y - 6 x)

Use differentiation rules

\frac{d}{d x} (x^{2}) + \frac{d}{d x} (y) + \frac{d}{d x} (- 6 x)

Use \frac{d}{d x} x^{n} = n x^{n - 1} to find derivative

2 x + \frac{d}{d x} (y) + \frac{d}{d x} (- 6 x)

Evaluate the derivative

More Steps

Evaluate

\frac{d}{d x} (y)

Use differentiation rules

\frac{d}{d y} (y) \times \frac{d y}{d x}

Use \frac{d}{d x} x^{n} = n x^{n - 1} to find derivative

\frac{d y}{d x}

2 x + \frac{d y}{d x} + \frac{d}{d x} (- 6 x)

Evaluate the derivative

More Steps

Evaluate

\frac{d}{d x} (- 6 x)

Use differentiation rule \frac{d}{d x} (c f (x)) = c \times \frac{d}{d x} (f (x))

- 6 \times \frac{d}{d x} (x)

Use \frac{d}{d x} x^{n} = n x^{n - 1} to find derivative

- 6 \times 1

Any expression multiplied by 1 remains the same

- 6

2 x + \frac{d y}{d x} - 6

2 x + \frac{d y}{d x} - 6 = \frac{d}{d x} (1)

Calculate the derivative

2 x + \frac{d y}{d x} - 6 = 0

Move the expression to the right-hand side and change its sign

\frac{d y}{d x} = 0 - (2 x - 6)

Subtract the terms

More Steps

Evaluate

0 - (2 x - 6)

If a negative sign or a subtraction symbol appears outside parentheses, remove the parentheses and change the sign of every term within the parentheses

0 - 2 x + 6

Removing 0 doesn't change the value,so remove it from the expression

- 2 x + 6

\frac{d y}{d x} = - 2 x + 6

Take the derivative of both sides

\frac{d}{d x} (\frac{d y}{d x}) = \frac{d}{d x} (- 2 x + 6)

Calculate the derivative

\frac{d ^{2} y}{d x ^{2}} = \frac{d}{d x} (- 2 x + 6)

Use differentiation rules

\frac{d ^{2} y}{d x ^{2}} = \frac{d}{d x} (- 2 x) + \frac{d}{d x} (6)

Evaluate the derivative

More Steps

Evaluate

\frac{d}{d x} (- 2 x)

Use differentiation rule \frac{d}{d x} (c f (x)) = c \times \frac{d}{d x} (f (x))

- 2 \times \frac{d}{d x} (x)

Use \frac{d}{d x} x^{n} = n x^{n - 1} to find derivative

- 2 \times 1

Any expression multiplied by 1 remains the same

- 2

\frac{d ^{2} y}{d x ^{2}} = - 2 + \frac{d}{d x} (6)

Use \frac{d}{d x} (c) = 0 to find derivative

\frac{d ^{2} y}{d x ^{2}} = - 2 + 0

Solution

\frac{d ^{2} y}{d x ^{2}} = - 2

Show Solution

Function

Solve the equation

Testing for symmetry

Identify the conic

Rewrite the equation

Find the first derivative

Find the second derivative

Graph